We prove that, for some suitable smooth bounded domain, there exists a solution to the following Neumann problem for the Lane-Emden system:

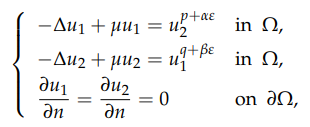
where $Ω$ is some smooth bounded domain in $\mathbb{R}^N,$ $N ≥ 4,$ $\mu > 0,$ $α > 0,$ $β > 0$ are constants and $ε\ne 0$ is a small number. We show that there exists a solution to the slightly supercritical problem for $ε > 0,$ and for $ε < 0,$ there also exists a solution to the slightly subcritical problem if the domain is not convex.
Comparing with the single elliptic equations, the challenges and novelty are manifested in the construction of good approximate solutions characterizing the boundary behavior under Neumann boundary conditions, in which process, the selection of the range of nonlinear coupling exponents and the weighted Sobolev spaces requires elaborate discussion.

The main purpose of this paper is to establish the distributional boundary values of functions in the weighted Hardy space, which improves the results of Carmichael in [4] and [8], where the weight function is linear. As our main result, we will prove that $f(z)$ in $H(ψ, Γ)$ has the $\mathcal{Z}'$ boundary value and can be expressed by the inverse Fourier transform of a distribution. Next, we will establish the $S'$ boundary value under stronger assumptions and give more precise expression if $f(z)$ also converges to $U ∈ D'_{L^p}(\mathbb{R}^n),$ where $1 ≤ p ≤ 2.$ In addition, we will also study the inverse result, in which we will prove that $f(z)$ is holomorphic on $T_Γ.$

In this paper, we mainly explore the existence of entire solutions of the quadratic trinomial partial differential-difference equation $$af^2(z)+2\omega f(z)(a_0f(z)+L^{k+s}_{1,2}(f(z)))+b(a_0f(z)+L^{k+s}_{1,2}(f(z)))^2=e^{g(z)}$$
by utilizing Nevanlinna’s theory in several complex variables, where $g(z)$ is entire functions in $\mathbb{C}^n,$ $ω\ne 0$ and $a, b, ω ∈ \mathbb{C}.$ Furthermore, we get the exact froms of solutions of the above differential-difference equation when $ω = 0.$ Our results are generalizations of previous results. In addition, some examples are given to illustrate the accuracy of the results.

We study the character of Thurston's circle packings with obtuse exterior intersection angles, and we get some simple criteria for the existence of hyperbolic circle packings. Moreover, the compactness theorem of combinatorial Ricci flows in hyperbolic background geometry with the weight function $Φ ∈ [0, π)$ is obtained. As a consequence, We generalize G. Lin's result [12] from acute exterior intersection angles case to obtuse exterior intersection angles case.