Cited by
This paper considers the solvability of boundary value problems with a $p$-Laplacian
By using the methods of upper and lower solution, the schäuder fixed point theorem, and the degree theory, we obtain the existence of one and triple solutions. This paper generalizes several problems due to the dependence on the $p$-Laplacian operator, the $n − 1$-th derivative not only in the differential equation but also in the boundary conditions. The most interesting point is that the solutions may be unbounded.
- BibTex
- RIS
- TXT
This paper considers the solvability of boundary value problems with a $p$-Laplacian
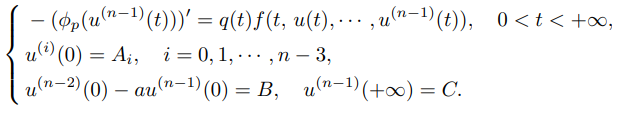
By using the methods of upper and lower solution, the schäuder fixed point theorem, and the degree theory, we obtain the existence of one and triple solutions. This paper generalizes several problems due to the dependence on the $p$-Laplacian operator, the $n − 1$-th derivative not only in the differential equation but also in the boundary conditions. The most interesting point is that the solutions may be unbounded.
}, issn = {}, doi = {https://doi.org/}, url = {http://global-sci.org/intro/article_detail/aam/18587.html} }This paper considers the solvability of boundary value problems with a $p$-Laplacian
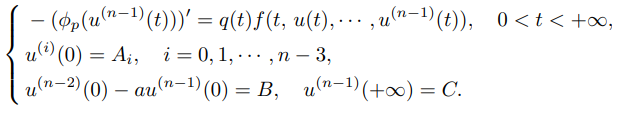
By using the methods of upper and lower solution, the schäuder fixed point theorem, and the degree theory, we obtain the existence of one and triple solutions. This paper generalizes several problems due to the dependence on the $p$-Laplacian operator, the $n − 1$-th derivative not only in the differential equation but also in the boundary conditions. The most interesting point is that the solutions may be unbounded.