- Journal Home
- Volume 37 - 2024
- Volume 36 - 2023
- Volume 35 - 2022
- Volume 34 - 2021
- Volume 33 - 2020
- Volume 32 - 2019
- Volume 31 - 2018
- Volume 30 - 2017
- Volume 29 - 2016
- Volume 28 - 2015
- Volume 27 - 2014
- Volume 26 - 2013
- Volume 25 - 2012
- Volume 24 - 2011
- Volume 23 - 2010
- Volume 22 - 2009
- Volume 21 - 2008
- Volume 20 - 2007
- Volume 19 - 2006
- Volume 18 - 2005
- Volume 17 - 2004
- Volume 16 - 2003
- Volume 15 - 2002
- Volume 14 - 2001
- Volume 13 - 2000
- Volume 12 - 1999
- Volume 11 - 1998
- Volume 10 - 1997
- Volume 9 - 1996
- Volume 8 - 1995
- Volume 7 - 1994
- Volume 6 - 1993
- Volume 5 - 1992
- Volume 4 - 1991
- Volume 3 - 1990
- Volume 2 - 1989
- Volume 1 - 1988
Cited by
In this paper, we study the asymptotic behavior of solutions to a quasilinear
fully parabolic chemotaxis system with indirect signal production and logistic source
under homogeneous Neumann boundary conditions in a smooth bounded domain $Ω⊂\mathbb{R}^n$ $(n ≥1)$, where $b ≥0$, $γ ≥1$, $a_i ≥1$, $µ$, $b_i >0$ $(i =1,2)$, $D$, $S∈ C^2([0,∞))$ fulfilling $D(s) ≥ a_0(s+1)^{−α}$, $0 ≤ S(s) ≤ b_0(s+1)^β$ for all $s ≥ 0,$ where $a_0,b_0 > 0$ and $α,β ∈ \mathbb{R}$ are constants. The purpose of this paper is to prove that if $b ≥ 0$ and $µ > 0$ sufficiently large, the globally bounded solution $(u,v,w)$ with nonnegative initial data $(u_0,v_0,w_0)$ satisfies $$\Big\| u(·,t)− \Big(\frac{b}{µ}\Big)^{\frac{1}{γ}}\Big\|_{L^∞(Ω)}+\Big\| v(·,t)−\frac{b_1b_2}{a_1a_2} \Big(\frac{b}{µ}\Big)^{\frac{1}{γ}} \Big\| _{L^∞(Ω)} +\Big\| w(·,t)−\frac{b_2}{a_2}\Big(\frac{b}{µ}\Big)^{\frac{1}{γ}}\Big\| _{L^∞(Ω)} →0$$ as $t→∞$.
- BibTex
- RIS
- TXT
In this paper, we study the asymptotic behavior of solutions to a quasilinear
fully parabolic chemotaxis system with indirect signal production and logistic source
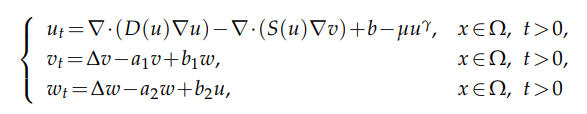
under homogeneous Neumann boundary conditions in a smooth bounded domain $Ω⊂\mathbb{R}^n$ $(n ≥1)$, where $b ≥0$, $γ ≥1$, $a_i ≥1$, $µ$, $b_i >0$ $(i =1,2)$, $D$, $S∈ C^2([0,∞))$ fulfilling $D(s) ≥ a_0(s+1)^{−α}$, $0 ≤ S(s) ≤ b_0(s+1)^β$ for all $s ≥ 0,$ where $a_0,b_0 > 0$ and $α,β ∈ \mathbb{R}$ are constants. The purpose of this paper is to prove that if $b ≥ 0$ and $µ > 0$ sufficiently large, the globally bounded solution $(u,v,w)$ with nonnegative initial data $(u_0,v_0,w_0)$ satisfies $$\Big\| u(·,t)− \Big(\frac{b}{µ}\Big)^{\frac{1}{γ}}\Big\|_{L^∞(Ω)}+\Big\| v(·,t)−\frac{b_1b_2}{a_1a_2} \Big(\frac{b}{µ}\Big)^{\frac{1}{γ}} \Big\| _{L^∞(Ω)} +\Big\| w(·,t)−\frac{b_2}{a_2}\Big(\frac{b}{µ}\Big)^{\frac{1}{γ}}\Big\| _{L^∞(Ω)} →0$$ as $t→∞$.
}, issn = {2079-732X}, doi = {https://doi.org/10.4208/jpde.v34.n2.3}, url = {http://global-sci.org/intro/article_detail/jpde/19184.html} }In this paper, we study the asymptotic behavior of solutions to a quasilinear
fully parabolic chemotaxis system with indirect signal production and logistic source
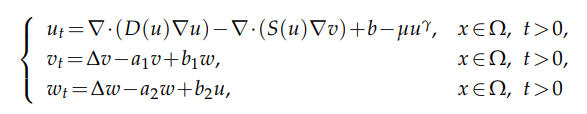
under homogeneous Neumann boundary conditions in a smooth bounded domain $Ω⊂\mathbb{R}^n$ $(n ≥1)$, where $b ≥0$, $γ ≥1$, $a_i ≥1$, $µ$, $b_i >0$ $(i =1,2)$, $D$, $S∈ C^2([0,∞))$ fulfilling $D(s) ≥ a_0(s+1)^{−α}$, $0 ≤ S(s) ≤ b_0(s+1)^β$ for all $s ≥ 0,$ where $a_0,b_0 > 0$ and $α,β ∈ \mathbb{R}$ are constants. The purpose of this paper is to prove that if $b ≥ 0$ and $µ > 0$ sufficiently large, the globally bounded solution $(u,v,w)$ with nonnegative initial data $(u_0,v_0,w_0)$ satisfies $$\Big\| u(·,t)− \Big(\frac{b}{µ}\Big)^{\frac{1}{γ}}\Big\|_{L^∞(Ω)}+\Big\| v(·,t)−\frac{b_1b_2}{a_1a_2} \Big(\frac{b}{µ}\Big)^{\frac{1}{γ}} \Big\| _{L^∞(Ω)} +\Big\| w(·,t)−\frac{b_2}{a_2}\Big(\frac{b}{µ}\Big)^{\frac{1}{γ}}\Big\| _{L^∞(Ω)} →0$$ as $t→∞$.