@Article{ATA-38-26,
author = {Ruggero Freddi , },
title = {Morse Index of Multiple Blow-up Solutions to the Two-Dimensional Sinh-Poisson Equation},
journal = {Analysis in Theory and Applications},
year = {2021},
volume = {38},
number = {1},
pages = {26--78},
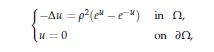
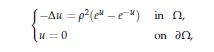
abstract = {<p style="text-align: justify;">In this paper we consider the Dirichlet problem</p><p style="text-align:center"><img src="https://admin.global-sci.org/uploads/ueditor/image/20211202/1638432534714476.jpg" title="1638432534714476.jpg" alt="2.JPG"/></p><p style="text-align: justify;">where $\rho$ is a small parameter and $\Omega$ is a $C^2$ bounded domain in $\mathbb{R}^2$. In [1], the author proves the existence of a $m$-point blow-up solution $u_\rho$ jointly with its asymptotic behaviour. We compute the Morse index of $u_\rho$ in terms of the Morse index of the associated Hamilton function of this problem. In addition, we give an asymptotic estimate for the first $4m$ eigenvalues and eigenfunctions.</p>},
issn = {1573-8175},
doi = {https://doi.org/10.4208/ata.OA-2020-0037},
url = {http://global-sci.org/intro/article_detail/ata/20011.html}
}